Nearpod version available
How Expensive are Payday Loans?
Objective
Students will be able to:
- Determine the total cost of a payday loan when given the amount financed, finance charge, and terms of the loan in weeks.
- Graph the relationship between the number of times a payday loan is renewed and the total cost of the loan.
- Rearrange the equation for the total cost of a payday loan to determine the finance charge.

In this personal finance lesson, students will learn how a payday loan works.
Resources
- Paper – blank sheets – one per group of 2-3 students
- Activity 1, one copy per student
- Activity 2, one copy per student
Activity 2 Key, answer guide - Activity 3, one copy per student
- FTC Payday loan video available at https://www.youtube.com/watch?v=WtruZLAiJkc Payday loan video (same video) also available here https://www.ftc.gov/media/70849
- Interactive graphing tool available at https://nces.ed.gov/nceskids/createagraph/
- Payday loan public service information available at https://consumer.ftc.gov/consumer-alerts/2020/04/what-you-should-know-about-payday-loans-and-car-title-loans
- CFPB payday loan information video https://www.youtube.com/watch?v=XUqyyJSoM_s&t=20s
- More information and FAQ’s on payday loans https://www.consumerfinance.gov/compliance/compliance-resources/consumer-lending-resources/payday-lending-rule/payday-lending-rule-faqs/
Procedure
Payday loans are used by consumers to meet short-term needs for cash. A typical two-week payday loan with a $15 per $100 fee equates to an annual percentage rate (APR) of almost 400%. The APR is the percentage of the principal of a loan to be paid as interest in one year and provides a way to compare loans. In this lesson, students will learn that many users of payday loans pay much more than they initially borrowed because of the costs of multiple renewals or rollovers. A rollover occurs when a borrower cannot repay the payday loan in full at the end of the term (usually two weeks) and then must continue the loan or take out a new one. Students will also use formulas to calculate the total cost of the loans and the APR.
- Ask students to imagine that they are adults. Tell them that their car needed to be inspected and learned that it needs $300 worth of repairs to pass inspection. Ask them what they would do if they didn’t have that amount of money saved. [Answers will vary but may include: borrow from a friend or family member, put it on a credit card, write a check and let it bounce, or wait to have the repairs done until the $300 is saved.]
- Tell the students that many people find themselves in these types of situations. Some people in this situation may choose to get cash by going to a payday lender and getting a payday loan. Draw a “K-W-L” (know, want to know, learned) chart on the board such as the one shown below.
| Know | Want to know | Learned |
|
|
- Ask students what they currently know about payday loans. Write these under the K/Know portion of the chart. Ask students what questions they have about payday loans. Record these responses under the W or Want to Know section of the chart. To elicit further responses, ask students what they think they might learn about payday loans from this lesson.
- Distribute Activity 1, one copy per student. Have students read the explanation of a payday loan. Discuss:
- What is a payday loan? [A payday loan is a loan issued to a borrower who writes a post-dated check made out to a lender, usually a company specializing in payday loans and other financial services targeted to low-income customers for the amount he or she wishes to borrow, plus a fee. The lender then gives the borrower cash in the amount stated on the check, minus the fee, and holds the check until the borrower’s next payday when the lender cashes it.]
- How much money do people usually borrow when getting a payday loan? [$500 or less]
- What is the finance charge? [The finance charge is the cost of taking out the loan. It may range from $10 to $30 for every $100 borrowed.]
- When do payday loans typically come due? [Your next payday – usually in two-weeks]
- What does rollover mean? [When a payday loan cannot be paid off in full when it is due, the borrower can renew or rollover the loan for another period – usually two weeks.]
- Return to the K/Know portion of the KWL chart on the board. Ask students:
- Did any of the information that you read verify what you said you knew about payday loans?[Answers will vary.]
- Did the reading give answers to any of the questions you had under the W/Want to Know section?[Answers will vary.] Record these under the L/Learned column.
- Tell students you are going to show them a video that explains how a typical payday loan works. Instruct them to pay careful attention to the rollovers.
- Show the video from the Federal Trade Commission available at https://www.youtube.com/watch?v=WtruZLAiJkc. If playing the video is not possible, read the transcript from Activity 3: Federal Trade Commission Resource Transcripts. The transcript can also be made available to students with oral processing challenges.
- Tell students that John – when faced with a problem similar to that posed to the class – chose to take out a payday loan. Ask students to explain whether they think John made a wise choice about how to pay for his truck repair. [Answers will vary.]
- Ask students what the total cost of John’s loan was. [$1,025] Ask them how they know that. [Answers will vary but will likely include “because the video told us.”]
- Tell them that together you are going to come up with several ways to determine the total cost of any payday loan. Ask students to suggest ways you can do this. [Possible answers: make a table or a graph, repeated addition, develop a formula] Depending on student responses, fill in the remaining methods in the answer.
- Tell students that first you are going to develop a table to solve the problem. Recreate the following table on the board:
| Weeks | Total Paid |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
- Ask students to provide the values for the “Weeks” column (0, 2, 4, 6, 8, 10, 12). Explain that the weeks start at zero because the first fee is paid when the loan is initiated. Since it is renewed or rolled over six times, the values are filled in by skip counting by two for the two weeks in each renewal or rollover.
| Weeks | Total Paid |
|
0 |
|
|
2 |
|
|
4 |
|
|
6 |
|
|
8 |
|
|
10 |
|
|
12 |
|
- Ask students how much the payday loan cost John when he first signed the paperwork (week zero). [$575 = $500 + $75 fee] Complete the first row of the table as shown below. Remind students that after two weeks, he couldn’t pay back the loan so he paid another $75 fee. Ask how much he had paid in all at this point? [$650] Complete the rest of the table by having students determine the total for each row.
| Weeks | Total Paid |
|
0 |
575 |
|
2 |
650 |
|
4 |
725 |
|
6 |
800 |
|
8 |
875 |
|
10 |
950 |
|
12 |
1025 |
- Ask students what the total cost of the loan would have been if he’d rolled it over for another two-week period (week 14). [$1,100]
- Use an interactive graphing tool on a graphing calculator or online to graph the relationship between the weeks and the total paid. One such tool is available from the National Center for Education Statistics at https://nces.ed.gov/nceskids/createagraph/. To obtain a graph such as the one below, follow the directions below for each tab. When facilitating with the class, ask students what changing the values does. Depending on the level of the students and the technology available, the graph can be completed as part of whole group instruction, in groups, or individually.


- Choose XY graph from the menu.
- Under the “design” tab, assign the following values and click update (XY type: line, background color: white, grid color: black, grid lines: 6, legend: no legend)
- Under the “data” tab, complete the following fields as shown in the graphic to the right and click update (graph title: Total Cost of a Payday Loan; X axis label: Weeks; y axis label: Total Cost in Dollars; data set points: 7; groups: 1; group label: blank; line width: medium; colors: green; point 1: 0, 575; point 2: 2, 650; point 3: 4, 725; point 4: 6, 800; point 5: 8, 875; point 6: 10, 950; point 7: 12, 1025; min-value: blank; max-value: blank)
- Click the “preview” tab.
The end result should appear as the one below. If desired, adjust the appearance of the graph by manipulating various aspects of the graph. In particular, adjust the minimum value of x to 0 and the maximum value of x to be 20 on the data tab. Update the graph and discuss the change. You can see more of the graph; however, the line does not extend. If you were graphing an equation for a line, it would continue. However, this is based solely on a set of data points.
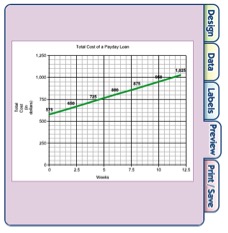
- Ask students to look at the graph and explain how they might estimate the total cost of the loan at a later time such as 16 weeks. [They can estimate the total cost of the loan at later dates by extending the graph and estimating the value.]
- Ask students if there is another way to calculate the total cost of a payday loan that wouldn’t take as long as setting up a table or creating a graph. [Develop an equation.]
- Divide the students into groups and ask them to develop a formula or equation for the total cost of a payday loan given the information they have available to them. For the purpose of standardized results, have the students use the following variables:
- Total cost = T
- Finance charge = F
- Loan amount = L
- Number of rollovers = R (point out the difference between using number of rollovers and number of weeks; i.e., four weeks = two rollovers).
- Allow several minutes for students to work on developing an equation. When most students have developed the equation or are at a point that they can’t get further in solving the problem without assistance, facilitate the development of the equation below using the responses generated during group work as your starting point. Write the following on the board:
Total cost = Loan amount + Finance charge(1 + Number of Rollovers)
or
T = L + F(1+R)
- Have students check their answer using various numbers of rollovers or “r” values from the example used previously. Remind students that the number of rollovers is the same as the number of weeks divided by two. One such example follows using four weeks or two rollovers.
Total cost = Loan amount + Finance charge(1 + Number of Rollovers)
T = L + F(1+R)
t = 500 + 75(1+2)
t = 500 + 75(3)
t = 500 + 225
t = 725
- Distribute Activity 2, one copy per student. Have students use the equation to solve the problems. Review student answers using Activity 2 Answer Key.
- Tell students that the federal government and others caution people against getting themselves into trouble by using expensive forms of credit such as payday loans. Tell them that they are going to act out a public service announcement with good advice for consumers from the Federal Trade Commission. Tell them to listen carefully and write down at least one alternative to a payday loan and one thing to consider when weighing one’s options.
- Read the transcript from Activity 3, Federal Trade Commission Resources Transcript and have two students act it out (one male and one female).
- Ask students for some alternatives people have to borrowing money – other than a payday loan. If necessary, replay the PSA telling students to listen carefully for these alternatives. [Take out a loan from a bank or credit union, ask for more time to pay the bill by talking to a creditor or credit counselor, apply money that is already saved, borrow money from family or friends, or use a credit card instead.]
- Tell students that the public service announcement also encourages consumers to compare the costs between their options. Ask students what features the PSA encouraged consumers to compare. [Annual percentage rate, fees, how soon the money must be repaid, what happens if you can’t repay the money]
- Distribute Activity 3, one copy per student. Have them review the transcript of the video and the PSA. Ask students the following:
- From the video, can we tell if John considered any options? [No.]
- If he had, which of these did he know?
- What is the annual percentage rate? [The video didn’t tell us.]
- What are the fees? [$75 for $500 borrowed.]
- How soon must he repay the money? [Two weeks]
- What happens if he can’t repay? [He must pay another $75 to renew or roll over the loan.]
- Point out that one very important piece of information was missing – the annual percentage rate or APR. Explain that the APR is the percentage cost of credit on an annual basis and the total cost of credit to the consumer, which includes any fees associated with the loan. It is the percentage of the principal of a loan to be paid as interest in one year. Interest is money paid, at a particular rate, for the use of borrowed money. Explain that in the United States the Truth in Lending Act requires all loans – including payday loans – to advertise the APR. The APR provides a way to compare loans.
- Tell students that there are formulas for calculating the APR.
APR = (finance charge/total amount financed) x (number of weeks in a year/number of weeks in term of loan) x 100
or
APR = (finance charge/total amount financed) x (365/number or days in term of loan) x 100
- Write the equation on the board as follows and solve for the APR:
APR = (finance charge/total amount financed) x (number of weeks in a year/number of weeks in term of loan) x 100
APR = (75/500) x (52/2) x 100
APR = .15 x 26 x 100
APR = 390%
Note: you can leave the x100 off in the equation, but you would need to convert your answer from a decimal (3.9) to a percent (390%).
- Provide additional practice if needed using the following problems:
- What is the APR on a payday loan in the amount of $600 with a finance charge of $60 per two weeks?
APR = (finance charge/total amount financed) x (number of weeks in a year/number of weeks in term of loan) x 100
APR = (60/600) x (52/2) x 100
APR = .1 x 26 x 100
APR = 260%
- Ethan borrows $700 from the payday lender for two weeks. The finance charge is $80. What is the APR?
APR = (finance charge/total amount financed) x (number of weeks in a year/number of weeks in term of loan) x 100
APR = (80/700) x (52/2) x 100
APR = .11 x 26 x 100
APR = 286%
- A friend is thinking about taking out a two-week payday loan to pay for a new set of tires that will cost $750. The finance charge will be $90. What is the APR?
APR = (finance charge/total amount financed) x (number of weeks in a year/number of weeks in term of loan) x 100
APR = (90/750) x (52/2) x 100
APR = .12 x 26 x 100
APR = 312%
- Ask students why the government might make reporting the APR on a payday loan – or any loan for that matter – a requirement. [This allows consumers to compare interest rates on the same basis – annual]
- Ask students where else they have heard of annual percentage rates and for the amount, if known. [Examples might include credit cards with APRs of 10% to 30%, car loans with APRs of 3% to 8%, student loans with APRs of 3% to 8%]
- Ask students what they can do to avoid being in a situation where they need money quickly and don’t have enough. [If the following answers are not provided, discuss each briefly: wise money management, saving money for emergencies, and establishing good credit.]
- Wrap up the lesson by revisiting the K-W-L chart created at the beginning of the lesson. If desired, watch this video from the CFFB https://www.youtube.com/watch?v=XUqyyJSoM_s&t=20s Ask students to share what they learned about payday loans from the lesson. Record student responses under the L/Learn portion of the chart.
- Review the following.
- What is a payday loan? [A payday loan – which might also be called a “cash advance” or “check loan” – is a short-term loan, generally for $500 or less, that is typically due on your next payday.]
- What is a finance charge? [A finance charge is the cost of taking out the loan.]
- What is a rollover? [When a payday loan cannot be paid off in full once it is due, the borrower can renew or roll over the loan for another period – usually two weeks.]
- What is interest? [The money paid for the use of borrowed money.]
- What is APR? [Annual percentage rate.]
- What are some alternatives to a payday loan for borrowing money? [Take out a loan from a bank or credit union, ask for more time to pay the bill by talking to a creditor or credit counselor, apply money that is already saved, borrow money from family/friends, or use a credit card instead.]
- Why do you think people take out payday loans? [Answers will vary but may include people needing cash quickly, it’s convenient—in the neighborhood; people don’t understand how payday loans work; or people don’t realize that there are alternatives.]
- Are payday loans ever a wise choice to get fast cash? [Answers will vary but most will say they are not a good source of fast cash.]
Assessment
Multiple Choice
- Which of the following is a characteristic of a payday loan?
- Loans are typically for amounts ranging from $500-$1,000
- Loans can be paid off in part or in full at any time
- [You need a checking account in order to get one]
- They are only used by people with bad credit
- Rasheem goes to a payday lender and borrows $450. He is told the finance charge will be $50 for two weeks. What is the total cost of his loan if it takes him six weeks to pay it off?
- $625
- [$650]
- $800
- $1,850
Constructed Response
Mary Ellen is comparing offers for payday loans from two different companies. She needs to borrow $600. While she knows both payday lenders loan money for two weeks at a time, she doesn’t feel that she will have the money to pay it off in just two weeks. She will pick up extra hours at work to save the money and hopes to pay it off in eight weeks. What would the total cost be at each lender? How much more expensive is the most expensive offer? Show your work.
Fast Cash advertises a finance charge of $90 for a $600 loan. Cash Now advertises a finance charge of $18 for every $100 you borrow.
[For each lender, the number of rollovers or R would be (8/2) or 4.]
Fast Cash:
Total cost = Loan amount + Finance charge (1 + Number of Rollovers)
T = L + F(1+R)
t = 600 + 90(1+4)
t = 600 + 90(5)
t = 600 + 450
t = $1,050
Cash Now:
Before using the formula, the finance charge also needs to be determined. Since she would borrow $600, the rate of $18 for every $100 borrowed needs to be calculated as (600/100)x18 = 6 x 18 = 108.
Total cost = Loan amount + Finance charge (1 + Number of Rollovers)
T = L + F(1+R)
t = 600 + 108(1+4)
t = 600 + 108(5)
t = 600 + 540
t = $1,140
Using Fast Cash would cost Mary Ellen $1,050 while using Cash Now would cost her $1,140. Cash Now would be $90 more expensive.

