
Grades 9-12

Don't have an account yet? Sign up for free
Don't have an account yet? Sign up for free
Nearpod version available
Students will be able to:

In this personal finance lesson, students will learn how to determine the function values of simple functions.
A government may tax a good or service in order to generate revenue. This will result in smaller producer and consumer surpluses and in dead weight loss. The tax burden is carried by the producer and consumer and can be calculated using different areas on the supply-demand graph for the good or service.
Mathematical straight line functions are used to calculate the corresponding price(s), (the y-value), asked and/or paid for a given quantity of a product, (the x-value). Equations to calculate the areas of rectangles and triangles are used to calculate different areas on the supply-demand graph.
[Answers may vary if a different equation and/or different values of x are used.]
|
|
x |
y |
4x – 3 = y |
Possible questions and answers |
|
1 |
0 |
-3 |
4(0) – 3 = 0-3 = – 3 |
Why is 4(0) = 0?
Multiplying by 0 always give 0
|
|
2 |
-1 |
-7 |
4(-1) – 3 = -4 – 3 = -7 |
Why is 4(-1) = -4?
Multiplying a negative number by a positive number always gives a negative answer. Why is -4-3 = -7? Adding 2 negative numbers will always give a negative answer. |
|
3 |
3 |
9 |
4(3) – 3 = 12 – 3 = 9 |
a. f(x) = 2/3(x) – 4 if x = 9
= 2/3(9) – 4
= 6 -4
= 2
b. 3y – 8x = 10 if x = – 2
3y – 8( -2) = 10
3y + 16 = 10
3y = 10 -16
y = -6/3
= – 2
Area of a Square: | X |
Area of a Rectangle: L X w
Area of a Triangle: (1/2)b + h
Area of a Circle: A=π(r)2; r is the radius
1. Area L X w
= 4 x 2.5
= 10
2. Area =(1/2)b x h
= ½ x 12 x 6
= 36
1. P = 5 – 2Q
P = 5- 2(1)
P = 3$
P = 5 – 2Q
0.5$ = 5 – 2Q
0.5 – 5 = -2Q
-4.5÷ -2 = Q
Q = 2.25 glasses
2. P = 2 + 1.5 Q
10 = 2 + 1.5 Q
(10-2) ÷1.5 = Q
Q = 5.3 burgers
P = 2 + 1.5 Q
12 = 2 + 1.5 Q
(12-2)÷1.5 = Q
Q = 6.6 burgers
| Question | Demand or supply card | Price and quantity card | Reason |
| 1 | Dark Blue “D” | Pink
“G” |
People buy fewer battery operated toys. This will result in decreased demand for these toys. The demand curve will, therefore, shift to the left
Price of the toys will decrease and the quantity exchanged will decrease. Change in taste and preferences. |
| 2 | Dark Green “B” | Yellow “F” | Wages go up—production costs up –supply decreases—supply graphs shifts left—price increases, quantity decreases
Change in cost of production. |
| 3 | Light Blue ”C” | White “E” | People buy more iPhones—more sim cards are bought—demand for sim cards increases—demand curve shits right – price increases, quantity increases
Change in the price of a complementary good. |
| 4 | Light Blue “C” | White “E” | Household gas can be used in place of coal. If the price of coal increases, less coal and more gas will be bought. The demand for gas will increase—the demand curve will shift to the right—price of gas will increase, quantity will increase.
Change in the price of a substitute. |
| 5 | Light Green “A” | Orange “H” | It is easier and cheaper to trap crabs. The supply of crabs will increase—more crab meat can be canned—the supply of canned crab meat will increase—the supply curve will shift to the right—price will decrease and quantity will increase.
Change in the cost of production. |
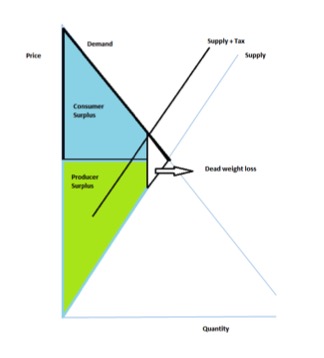
A.
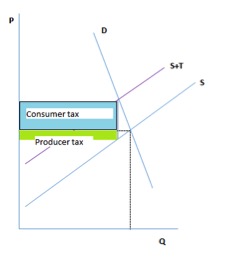
In this case, the consumer will bear a larger part of the tax burden.
B.
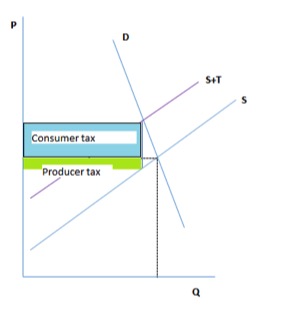
In this case, the consumer will bear a larger part of the tax burden.
C.
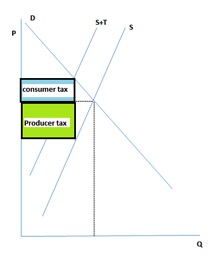
In this case, the consumer will bear a larger part of the tax burden.
|
Equation |
y |
x |
Calculation |
| y = -3 x |
|
2/3 |
y = -3 (2/3) = -2 |
| y = 4 x – 6 |
|
-2 | y = 4(-2) – 6 = -14 |
| y = 2 x + 3 |
5 |
|
5 = 2 x + 3
5-3 = 2 x 2÷2 = x x = 1 |
Multiple Choice
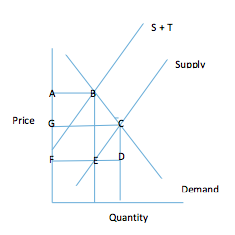
Constructed Response
You are the newly appointed Head Economist of Super Health Food Industries. Your first task is to evaluate the present situation regarding the supply and demand of one of the company’s most popular products: The X-Factor Apple Sauce.
Presently the price is $3 per bottle of 330 ml and five million bottles are sold per month.
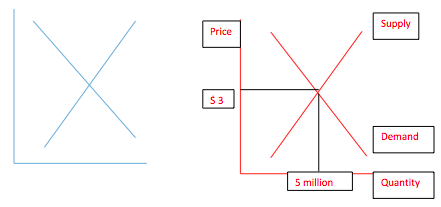
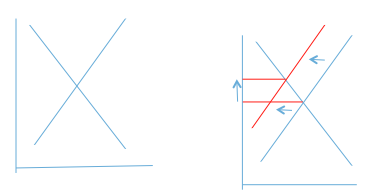
Price will rise, quantity exchanged with fall.
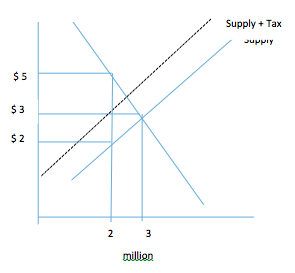
Answer: producer tax burden: 2 million bottles x $1 = $2 million.
½ x (1 million) x $1 = $1/2 million.
The producers’ incidence of this tax is $1 per bottle.
Consumer tax burden: 2 million bottles x $2 = $4 million.
½ x (1 million) x$2 = $1 million.
Consumers’ incidence of this tax is $2 per bottle.
The consumers effectively pay $2 of this tax and the producers effectively pay $1 of the tax per bottle.
Dead weight loss: ½ x $3 x 1 million bottles: $ 1 ½ million.

Grades 9-12

Grades 9-12

Grades 9-12

Grades 9-12
